I want to ask whether Hooke’s law formula F=kx holds as it is for conical compression spring or with some modifications.
Secondly, which standards or book do you recommend for complete understanding of design and formulas for conical compression springs?
Dear Mr. Farhan,
Thank you for your question. With conical springs, there is no concept of the spring constant (k) after the spring reaches its solid height because it changes. However, there is a general formula to determine loads of conical springs. Please find attached the formula.
If you have any other questions, please let us know anytime.
Best regards,
Tokai Spring’s Support Team
Attachments:
The tapered springs obey Hooke’s over the widest range of loads when suspended with the large diameter end down. This is why they are made that way. Explain why this works.
Thank you for your reply.
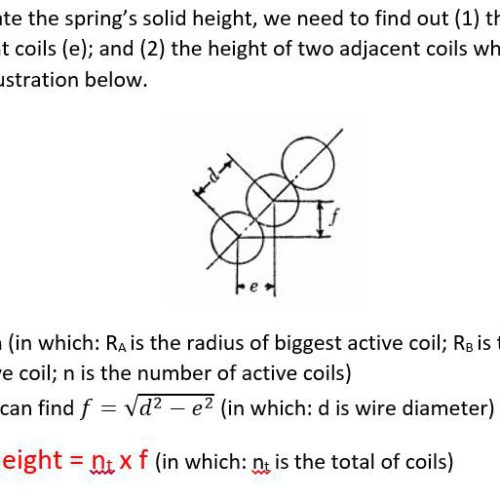
How can we calculate solid length of a conical compression spring? As for cylindrical compression springs Lf=Nt x d
Where Lf= Free length
Nt= Total coils
d= wire dia
Dear Mr. Farhan,
Our apologies for the late reply. Please find attached our answer to your question.
We hope you find it helpful. If you have any other questions, please let us know.
Best regards,
Tokai Spring’s Support Team
Attachments:
1) Hooke’s over the widest range of loads when is why they are made that way. Explain why this works.
2) some springs are wound with the wire under torsion, so that they are naturally tightly compressed. The plot of extension vs. Applied force will show intercept on the extension axis is negative, that is the ‘unstreched length’ is shorter than the actual unloaded length. Explain.
Attachments:
Dear Charles,
Please find our answers to your questions as follows.
①
We will answer with the general characteristics of conical springs.
When the conical springs are used with the maximum diameter down and compressed from the minimum diameter, the coils on the minimum diameter side fit inside the maximum diameter as the compression increases.
Comparing a cylindrical coil spring and a conical spring with the same three elements: wire diameter, free length, and the number of coils, the conical spring is able to compress more until the solid length for the above reason.
Depending on the shape, there are even conical springs with shapes that allow them to be compressed to a length where the solid length equals the wire diameter.
The relationship between load and deflection of conical springs follows Hooke’s law P (load) = k (spring constant) × δ (deflection), but it is not a linear relationship like normal cylindrical coil springs.
The reason is that when being compressed, the coil diameter of the effective part becomes smaller, and the number of active coils also decreases, so the spring constant becomes stronger.
②
We will answer this question with the characteristics of tightly coiled extension springs with initial tension.
They are extension springs that are coiled and twisted in the direction opposite the twisting direction applied to the wire during use.
The result is an initial tension.
The initial tension is the force that works in the direction toward the solid-height state even when the springs are in the free state. Therefore, even if the extension springs are pulled, the length of the springs remains unchanged until the initial tension and the force are balanced.
For the relationship between load and deflection, please refer to Graph 7 on this site.
The X-axis is the deflection and the Y-axis is the force. As you can see, even if the X-axis (deflection) is 0 mm, the linear relationship starts from the point where it intersects the positive side axis with the Y-axis (force) (intercept is positive).
This is not possible in practice, but if this linear characteristic is extended to the minus side of the X-axis (deflection), the intercept with the X-axis (deflection) will be negative.
In other words, assuming a load of 0N, the deflection is negative, this can be rephrased as “shorter than the actual free length”.
Best regards,
Tokai Sring’s Support Team
Why does the tampered springs obey hooke’s over the widest range of loads when suspended with the large diameter end down
Dear Folorunso,
Thank you for the question.
We will answer with the general characteristics of conical springs.
When the conical springs are used with the maximum diameter down and compressed from the minimum diameter, the coils on the minimum diameter side fit inside the maximum diameter as the compression increases.
Comparing a cylindrical coil spring and a conical spring with the same three elements: wire diameter, free length, and the number of coils, the conical spring is able to compress more until the solid length for the above reason.
Depending on the shape, there are even conical springs with shapes that allow them to be compressed to a length where the solid length equals the wire diameter.
The relationship between load and deflection of conical springs follows Hooke’s law P (load) = k (spring constant) × δ (deflection), but it is not a linear relationship like normal cylindrical coil springs.
The reason is that when being compressed, the coil diameter of the effective part becomes smaller, and the number of active coils also decreases, so the spring constant becomes stronger.
We hope this helps.
Best regards,
Tokai Spring’s Support Team
Pls help me solve number 3
Attachments:
Thank you for your question!
However, with the above information, we can not understand the meaning of the question.
It would be much appreciated if you could explain more so that we can support you.
Best regards,
Tokai Spring’s Support Team
Why does tapered spring obey hookes law when wide range of loads are suspended with the large diameter end down.
Dear Pleasant,
Thank you for your question!
We will answer with the general characteristics of conical springs.
When the conical springs are used with the maximum diameter down and compressed from the minimum diameter, the coils on the minimum diameter side fit inside the maximum diameter as the compression increases.
Comparing a cylindrical coil spring and a conical spring with the same three elements: wire diameter, free length, and the number of coils, the conical spring is able to compress more until the solid length for the above reason.
Depending on the shape, there are even conical springs with shapes that allow them to be compressed to a length where the solid length equals the wire diameter.
The relationship between load and deflection of conical springs follows Hooke’s law P (load) = k (spring constant) × δ (deflection), but it is not a linear relationship like normal cylindrical coil springs.
The reason is that when being compressed, the coil diameter of the effective part becomes smaller, and the number of active coils also decreases, so the spring constant becomes stronger.
We hope it helps!
Best regards,
Tokai Spring’s Support Team
